Electrostatic approximation[edit]
The validity of the electrostatic approximation rests on the assumption that the electric field is irrotational:
From Faraday's law, this assumption implies the absence or near-absence of time-varying magnetic fields:
In other words, electrostatics does not require the absence of magnetic fields or electric currents. Rather, if magnetic fields or electric currentsdo exist, they must not change with time, or in the worst-case, they must change with time only very slowly. In some problems, both electrostatics and magnetostatics may be required for accurate predictions, but the coupling between the two can still be ignored. Electrostatics and magnetostatics can both be seen as Galinean limits for electromagnetism.[2]
Electrostatic potential[edit]
Because the electric field is irrotational, it is possible to express the electric field as the gradient of a scalar function, , called the electrostatic potential (also known as the voltage). An electric field,
, called the electrostatic potential (also known as the voltage). An electric field,  , points from regions of high electric potential to regions of low electric potential, expressed mathematically as
, points from regions of high electric potential to regions of low electric potential, expressed mathematically as
 , called the electrostatic potential (also known as the voltage). An electric field,
, called the electrostatic potential (also known as the voltage). An electric field,  , points from regions of high electric potential to regions of low electric potential, expressed mathematically as
, points from regions of high electric potential to regions of low electric potential, expressed mathematically as
The Gradient Theorem can be used to establish that the electrostatic potential is the amount of work per unit charge required to move a charge from point  to point
to point  is the following line integral:
is the following line integral:
 to point
to point  is the following line integral:
is the following line integral:
From these equations, we see that the electric potential is constant in any region for which the electric field vanishes (such as occurs inside a conducting object).
Electrostatic energy[edit]
Main articles: Electric potential energy and Energy density
A single test particle's potential energy,  , can be calculated from a line integral of the work,
, can be calculated from a line integral of the work,  . We integrate from a point at infinity, and assume a collection of
. We integrate from a point at infinity, and assume a collection of 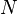 particles of charge
particles of charge  , are already situated at the points
, are already situated at the points  . This potential energy (in Joules) is:
. This potential energy (in Joules) is:
 , can be calculated from a line integral of the work,
, can be calculated from a line integral of the work,  . We integrate from a point at infinity, and assume a collection of
. We integrate from a point at infinity, and assume a collection of 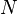 particles of charge
particles of charge  , are already situated at the points
, are already situated at the points  . This potential energy (in Joules) is:
. This potential energy (in Joules) is:
where  is the distance of each charge
is the distance of each charge  from the test charge
from the test charge  , which situated at the point
, which situated at the point 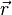 , and
, and  is the electric potential that would be at
is the electric potential that would be at 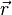 if the test charge were not present. If only two charges are present, the potential energy is
if the test charge were not present. If only two charges are present, the potential energy is  . The total electric potential energy due a collection of N charges is calculating by assembling these particles one at a time:
. The total electric potential energy due a collection of N charges is calculating by assembling these particles one at a time:
 is the distance of each charge
is the distance of each charge  from the test charge
from the test charge  , which situated at the point
, which situated at the point 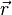 , and
, and  is the electric potential that would be at
is the electric potential that would be at 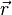 if the test charge were not present. If only two charges are present, the potential energy is
if the test charge were not present. If only two charges are present, the potential energy is  . The total electric potential energy due a collection of N charges is calculating by assembling these particles one at a time:
. The total electric potential energy due a collection of N charges is calculating by assembling these particles one at a time:
where the following sum from, j = 1 to N, excludes i = j:
This electric potential,  is what would be measured at
is what would be measured at  if the charge
if the charge  were missing. This formula obviously excludes the (infinite) energy that would be required to assemble each point charge from a disperse cloud of charge. The sum over charges can be converted into an integral over charge density using the prescription
were missing. This formula obviously excludes the (infinite) energy that would be required to assemble each point charge from a disperse cloud of charge. The sum over charges can be converted into an integral over charge density using the prescription  :
:
 is what would be measured at
is what would be measured at  if the charge
if the charge  were missing. This formula obviously excludes the (infinite) energy that would be required to assemble each point charge from a disperse cloud of charge. The sum over charges can be converted into an integral over charge density using the prescription
were missing. This formula obviously excludes the (infinite) energy that would be required to assemble each point charge from a disperse cloud of charge. The sum over charges can be converted into an integral over charge density using the prescription  :
: ,
,
This second expression for electrostatic energy uses the fact that the electric field is the negative gradient of the electric potential, as well asvector calculus identities in a way that resembles integration by parts. These two integrals for electric field energy seem to indicate two mutually exclusive formulas for electrostatic energy density, namely  and
and  ; they yield equal values for the total electrostatic energy only if both are integrated over all space.
; they yield equal values for the total electrostatic energy only if both are integrated over all space.
 and
and  ; they yield equal values for the total electrostatic energy only if both are integrated over all space.
; they yield equal values for the total electrostatic energy only if both are integrated over all space.Electrostatic pressure[edit]
On a conductor, a surface charge will experience a force in the presence of an electric field. This force is the average of the discontinuous electric field at the surface charge. This average in terms of the field just outside the surface amounts to:
 ,
,
This pressure tends to draw the conductor into the field, regardless of the sign of the surface charge.
rest continue in part 4.







No comments:
Post a Comment