Electric field
Electric field lines are useful for visualizing the electric field. Field lines begin on positive charge and terminate on negative charge. Electric field lines are parallel to the direction of the electric field, and the density of these field lines is a measure of the magnitude of the electric field at any given point. The electric field,  , (in units of voltsper meter) is a vector field that can be defined everywhere, except at the location of point charges (where it diverges to infinity). It is convenient to place a hypothetical test charge at a point (where no charges are present). By Coulomb's Law, this test charge will experience a force that can be used to define the electric field as follow
, (in units of voltsper meter) is a vector field that can be defined everywhere, except at the location of point charges (where it diverges to infinity). It is convenient to place a hypothetical test charge at a point (where no charges are present). By Coulomb's Law, this test charge will experience a force that can be used to define the electric field as follow
 , (in units of voltsper meter) is a vector field that can be defined everywhere, except at the location of point charges (where it diverges to infinity). It is convenient to place a hypothetical test charge at a point (where no charges are present). By Coulomb's Law, this test charge will experience a force that can be used to define the electric field as follow
, (in units of voltsper meter) is a vector field that can be defined everywhere, except at the location of point charges (where it diverges to infinity). It is convenient to place a hypothetical test charge at a point (where no charges are present). By Coulomb's Law, this test charge will experience a force that can be used to define the electric field as follow
(See the Lorentz equation if the charge is not stationary.)
Consider a collection of 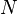 particles of charge
particles of charge  , located at points
, located at points  (called source points), the electric field at
(called source points), the electric field at 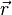 (called the field point) is:
(called the field point) is:
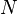 particles of charge
particles of charge  , located at points
, located at points  (called source points), the electric field at
(called source points), the electric field at 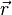 (called the field point) is:
(called the field point) is:
where  is the displacement vector from a source point
is the displacement vector from a source point to the field point
to the field point 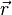 , and
, and  is a unit vector that indicates the direction of the field. For a single point charge at the origin, the magnitude of this electric field is
is a unit vector that indicates the direction of the field. For a single point charge at the origin, the magnitude of this electric field is  and points away from that charge is positive. That fact that the force (and hence the field) can be calculated by summing over all the contributions due to individual source particles is an example of the superposition principle. The electric field produced by a distribution of charges is given by the volume charge density
and points away from that charge is positive. That fact that the force (and hence the field) can be calculated by summing over all the contributions due to individual source particles is an example of the superposition principle. The electric field produced by a distribution of charges is given by the volume charge density  and can be obtained by converting this sum into a triple integral:
and can be obtained by converting this sum into a triple integral:
 is the displacement vector from a source point
is the displacement vector from a source point to the field point
to the field point 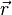 , and
, and  is a unit vector that indicates the direction of the field. For a single point charge at the origin, the magnitude of this electric field is
is a unit vector that indicates the direction of the field. For a single point charge at the origin, the magnitude of this electric field is  and points away from that charge is positive. That fact that the force (and hence the field) can be calculated by summing over all the contributions due to individual source particles is an example of the superposition principle. The electric field produced by a distribution of charges is given by the volume charge density
and points away from that charge is positive. That fact that the force (and hence the field) can be calculated by summing over all the contributions due to individual source particles is an example of the superposition principle. The electric field produced by a distribution of charges is given by the volume charge density  and can be obtained by converting this sum into a triple integral:
and can be obtained by converting this sum into a triple integral:

- The electrostatic field (lines with arrows) of a nearby positive charge (+)causes the mobile charges in conductive objects to separate due to electrostatic induction. Negative charges (blue) are attracted and move to the surface of the object facing the external charge. Positive charges (red) are repelled and move to the surface facing away. These induced surface charges are exactly the right size and shape so their opposing electric field cancels the electric field of the external charge throughout the interior of the metal. Therefore the electrostatic field everywhere inside a conductive object is zero, and the electrostatic potential is constant.
Gauss's law[edit]
Gauss's law states that " the total electric flux through any closed surface in free space of any shape drawn in an electric field is proportional to the total electric charge enclosed by the surface." Mathematically, Gauss's law takes the form of an integral equation:
where  is a volume element. If the charge is distributed over a surface or along a line, replace
is a volume element. If the charge is distributed over a surface or along a line, replace  by
by  or
or  . The Divergence Theorem allows Gauss's Law to be written in differential form:
. The Divergence Theorem allows Gauss's Law to be written in differential form:
 is a volume element. If the charge is distributed over a surface or along a line, replace
is a volume element. If the charge is distributed over a surface or along a line, replace  by
by  or
or  . The Divergence Theorem allows Gauss's Law to be written in differential form:
. The Divergence Theorem allows Gauss's Law to be written in differential form:
where  is the divergence operator.
is the divergence operator.
 is the divergence operator.
is the divergence operator.Poisson and Laplace equations[edit]
The definition of electrostatic potential, combined with the differential form of Gauss's law (above), provides a relationship between the potential Φ and the charge density ρ:
This relationship is a form of Poisson's equation. In the absence of unpaired electric charge, the equation becomes Laplace's equation:
now rest continue in part 3.






No comments:
Post a Comment